
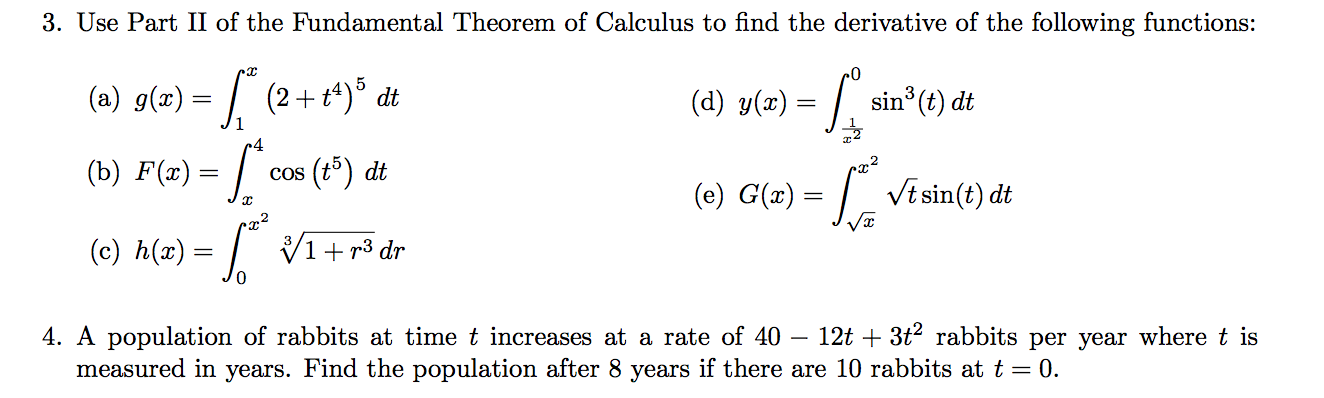
Part 1 of the fundamental theorem of calculus is used to differentiate an integral. What is the Fundamental Theorem of Calculus Part 1 Formula?

FTC 2: ∫ a b f(x) dx = F(b) - F(a) where F(x) = ∫ f(x) dx.The fundamental theorem of calculus (FTC) tells us the relationship between derivatives and integrals. (d/dx) ∫ x 2x cos t 2 dt = - cos x 2 + 2 cos (4x 2)Īnswer: (d/dx) ∫ x 2x cos t 2 dt = 2 cos (4x 2) - cos x 2.įAQs on Fundamental Theorem of Calculus What are Two Parts of the Fundamental Theorem of Calculus? (d/dx) ∫ 0 2x cos t 2 dt = d/dx ∫ 0 u cos t 2 dt To evaluate the second derivative (which is (d/dx) ∫ 0 2x, let us assume that u = 2x. The value of the first derivative directly follows from FTC 1. Now we will take the derivative on both sides.ĭ/dx ∫ x 2x cos t 2 dt = - (d/dx) ∫ 0 x cos t 2 dt + (d/dx) ∫ 0 2x cos t 2 dt. ∫ x 2x cos t 2 dt = ∫ x 0 cos t 2 dt + ∫ 0 2x cos t 2 dt Using the properties of definite integrals, we can write the given integral as follows. Let us recall the first part of the fundamental theorem of calculus (FTC 1) which says d/dx ∫ a x f(t) dt = f(x). Hence the second fundamental theorem of integral calculus is proved.Įxample 2: Evaluate the following derivative of the integral: (d/dx) ∫ x 2x cos t 2 dt. ∫ a b f(t) dt - F(b) = ∫ a a f(t) dt - F(a)īy a property of definite integrals, ∫ a a f(t) dt = 0. Thus, h(x) is a constant function over and hence We know that h(x) is continuous on (as both g(x) and F(x) are continuous on the same interval) and from the above equation h'(x) = 0. Let us define another function h(x) such that

Then by the first part of the fundamental theorem of calculus (FTC 1), g'(x) = f(x). Let us define a new function g(x) such that
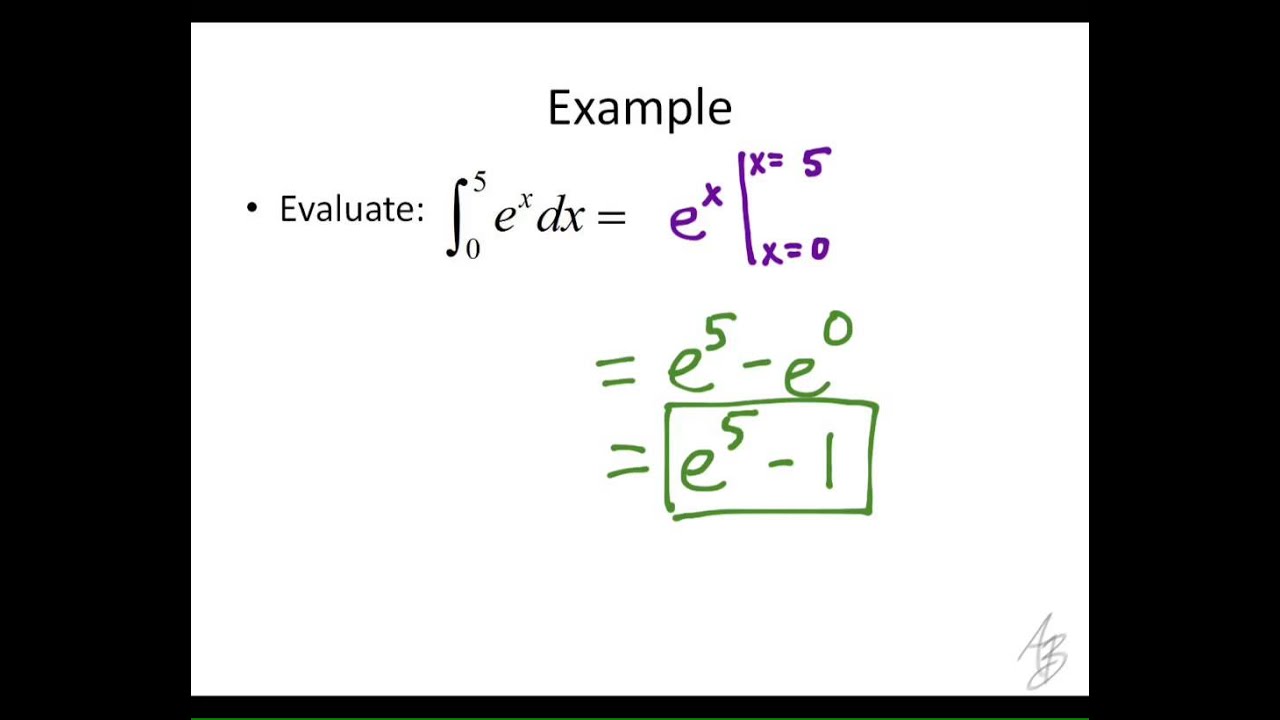
It is given that F(x) is an anti derivative of f(x). "If f(x) is a continuous function over and if F(x) is some antiderivative of f(x) (i.e., F'(x) = f(x)) then ∫ a b f(x) dx = F(b) - F(a)".The second fundamental theorem of calculus (FTC 2) is stated as follows. This theorem helps to evaluate a definite integral without using the Riemann sum (or calculating the area under the curves). Usually, to calculate a definite integral of a function, we will divide the area under the graph of that function lying within the given interval into many rectangles and then we add the areas of all such rectangles (this process is named as Riemann integration). The second fundamental theorem of calculus (FTC Part 2) says the value of a definite integral of a function is obtained by substituting the upper and lower bounds in the antiderivative of the function and subtracting the results in order. Second Fundamental Theorem of Calculus (Part 2) Hence the first fundamental theorem of calculus is proved. Since f(x) is continuous on and since c is also present in this interval, by the definition of continuity, (Recalling mean value theorem: If f(x) is continuous on, then there exists atleast some point c in such that f(c)= ∫ a b f(x) dx) Since f(x) is continuous on (this is because f(x) is continuous on and is a subinterval of ), by mean value theorem, there exists at least one point c in the interval such that, Using this in the above equation,į'(x) = lim h → 0 (1/h) ∫ x x+h f(t) d t. Using this in the above equation,īy another property of definite integrals, ∫ a b f(x) dx + ∫ b c f(x) dx = ∫ a c f(x) dx. Using this definition in the above equation,į'(x) = lim h → 0 (1/h) īy a property of definite integrals, ∫ a b f(x) dx = - ∫ b a f(x) dx. "If f(x) is a function that is continuous over and differentiable over (a, b) and if F(x) is defined as F(x) = ∫ a x f(t) dt then F'(x) = f(x) over the interval " (OR)īy the definition of the derivative of a function,.The first fundamental theorem of calculus (FTC 1) is stated as follows. Using this theorem, we can evaluate the derivative of a definite integral without actually evaluating the definite integral. The first fundamental theorem of calculus (FTC Part 1) is used to find the derivative of an integral and so it defines the connection between the derivative and the integral. First Fundamental Theorem of Calculus (Part 1)


 0 kommentar(er)
0 kommentar(er)
